Presentation: Symmetry

Topic: Symmetry

Instruction:

1- Each student is given a sheet of paper of different shape
2- Find a partner (any student in the classroom) of the same shape as yours.
3- As a whole class, use the shape that you have to come up with this:

Note:

1- Symmetry is a big word for primary school students.
2- Therefore, they need to learn it by hands-on activity.
3- This activity is to introduce the concept of symmetry, or to reinforce students' memory on shapes

Statistics and Probability

Statistics refers to the process of collection, organization and interpretation of data. During the workshop, I was using Smarties to understand this concept. The data that I had collected was based on the colour of Smarties that each student has.


Firstly, I collected from each person the number of Smarties that they have according to color. There are pink, blue, yellow, green, red, brown and purple.


Secondly, based on the data collected, I knew the exact amount of each colour and I drew a bar graph on the magic board.


Finally, I was able to make some interpretation such as green and red colour have the most amount of all the colour and pink is the fewest. Whereas, blue and purple share the same amount.






Probability is the number of ways that an event can occur.


An example of probability. At the end, all has the same probability to reach which is 1.

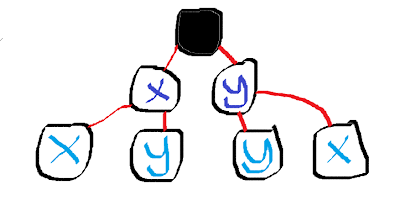
                                 (A) X-X         (B) X-Y           (C) Y-Y      (D) Y-X  

This second example display how at the end they have a different probibility.

                                      (A) X-X          (B) X-Y, Y-Y     (C) Y-X

(I regret that I did not capture even one photo of what I had done in the workshop. )

Geometry

Geometry is a branch of mathematics concerned with subject of shape, size, space and position. Points, line, symmetry and circle are the common things found when this subject is discussed.


Shape


There are many objects in our life that have similar shapes. Books, dictionaries, televisions, computers and papers are of the same shape - rectangle. Balls, water melons, oranges and tomatoes are round. Water bottle and bananas are cylinder. 


During the workshop with Anne, we had come up with a village using papers. We created road, houses, buildings and junction using papers by designing many different shapes. 

the village in the making..

Symmetry

When a shape can be folded to become another shape, that is symmetry. For example, a rectangle can be folded into two shape. Thus, we consider a rectangle to have two symmetry. 

In the workshop, we had the opportunity to try finding how many symmetry the shapes have using a mirror. If the mirror reflects the same shape, so it is symmetry.

Symmetry is a big word for young learners, therefore the introduction of the word should be accompanied by interesting activities. There are many suggestion of activities that teachers can carry out here

Tangrams

Tangram is a puzzle consisting seven flat shapes which can be put together to form shapes. The shapes are rectangle, triangle, parallelogram and trapezium. 

a parallelogram 

a triangle

a rectangle

Teacher tip-tops:

1- This topic really needs to be done using hands-on activity or games. There is no point for teachers to put more emphasis on explaining the meaning of any words used in the topic. Thus, teacher should prepare a range of activity to reinforce students' understanding.

2- Paper is the most suitable material to cater for all students' need in a lesson of this topic. Students can explore the concept of shape, symmetry and tangram using paper.

Measurement: Standard Unit

The second part of measurement is to use standard unit measurement. Standard unit measurement refers to definite magnitude of a physical quantity defined by conventional law and used as a standard measurement by people. 

We, people, engage with lot of standard unit measurement in our everyday life. For instance, using money at shopping mall, weighting our weight in the gymnasium, determining the volume of ingredient we put when we bake a cake and look for the distance between our current location and destination that we head to.

measurement is important in preparing the ingredient to bake a cake

Given all these situations, it is no doubt that children should be exposed to the concept of measurement in school. 

Standard unit measurement encapsulate concept of mass, weight, time, distance, length, weight, height, volume, area, perimeter and money. Each concept has different measurement units and measurement materials. 

For example, we use meter, centimeter and millimeter for length and perimeter. For time, there are seconds, minutes and hour or even further day, week, fortnight, month, year and decade. Gram, kilogram and milligrams are the units for mass.

today, almost every house own this to measure their weight  

this is normally used by tailor to measure their customers' body parts

ruler, very commonly used by students in school

Teacher tip-tops:

1- To have an understanding, repetition is important but the challenge is to make the repetition not a boredom. Off course, students will find it redundant to measure the same thing in the classroom. Thus, teachers' creativity is needed to provide students with array of opportunity to practice. For example, getting students to work outside the classroom or with people around the school. For example, ask the students to get weight of 20 people or measure the area of many places around the school. 

2- Again, teachers should not limit students' curiosity to explore on their own by giving too many rules and instruction. Task-based learning can be employed in giving the students opportunity to 'play' with the concept. The tasks should be made to be general so that students can think on their own. For example, teachers ask the students to come up with ten function of a ruler and show how they use it.

Measurement: Non-standard

Measurement is the process of estimating or determining the quantitative value of any objects. Measurement is always connoted with mass, weight, height, volume and area. The ability of children to use the specific measuring items should be preceded by the ability to measure using things around their life. 


Non standard measurement before standard measurement


Non standard measurement advocates the manipulation of classroom materials, natural material, body parts or classroom furniture to measure. This type of measurement should emphasize the development of comparative language such as 'more than', 'less than', 'bigger/ smaller than' and 'taller/ shorter than'.

 

is bigger than 

This reflects the way people in the olden days (hundred years ago) measure things. They were manipulating their feet and hand to measure the area of their land, using the gap between sunrise and sunset to know how long they had been to work or even using containers of the same size to divide things among them. 

using feet to measure length of an object e.g. land, wall, floor, etc.

In the workshop with Anne, we had the opportunity to measure many things using non-standard unit. 


For example, using the area of a table to measure the area of the white board. We were using butch papers to represent the table and pasting the papers on the white boards. Finally, we discovered that the white board is equal to 12 tables.


We also tried to measure our body parts - height, hand, body and nose - using paper clips, unifix cubes, paddle pop sticks, straws. It was interesting to know the difference of measurement among us.


Also, we use pendulum to measure the time to construct a unifix tower of ten cubes. We found out that the times taken to completely construct a unifix tower differ as the length of the string changes.

use pendulum to measure time taken for an activity

Teacher tip-tops:


1- In setting up the area for teaching and learning measurement, teachers can either have one area to store all the materials in measurement or different areas for different types of materials. Also, restoring some materials of different types in a box is also interesting so that students can bring the box to where they want to measure things (especially outside the classroom). It works like a kit box.


2- Provision of opportunity for students to self explore the measurement materials before directed activities. However, guidance is still a necessity by giving questions like "I wonder if..." or "How many ways..". If students are able to produce or bring or think of an item to be measurement material, they should be used in classroom and shared among the class.


3- Directed activities are still prevalent but without too many instruction and rules which can limit their creativity and curiosity. Teachers should always give time for the students to explore and understand the concepts on their own as this will result in great insights. 

Algebra: Balance

Do you know how object in the picture below works?

 

This is a scale. This object functions to measure the weight of any object. Back then, we found this a lot in shops to measure the weight of items that they sell. It employs the concept of balancing to know the weight.

In algebra, we have a term called balancing. Balancing refers to the process in which we attempt to balance two values. In mathematics, we use a lot of equation which obviously employ this concept. For example, 3x + 5 = 9 + 2x. The symbol '=' indicates that the first part (3x + 5) and the second part (9 = 2x) share the same value. 

The understanding of this concept can assist young learners in accomplishing addition and subtraction problems, or any mathematical problems. 

In the workshop, we 'played' with this object to visualize how we can solve addition and subtraction using balancing concept. Balancing concept claims that the two parts of the object should be in the same value or weight so that it will be balanced. Therefore, if we have 10 for one part, the other part should have ten. 

For example, the question is:


4 + __ = 10


By understanding the concept of balancing, students can work on finding the question. On one part, they put the weight to be 10 'value' and the other part 4 'value'. So they have to count how many more 'value' to balance the scale.


Using this concept also help students knowing lots of ways to reach


   1  2  3  4  5  6  7  8  9      I       9  8  7  6  5  4  3  2  1
             part A              middle             part B




For example, if the students discover that in part A they have two fives (5 + 5), they should be able to reach to another equation of the same value. By retaining the value in part A and moving the value in part B, they should know that 4 + 6, 3 + 7, 2 + 8 and 1 + 9 are equal to 5 + 5.

Algebra: Function

Have you ever watched a magic show? There are times in a magic show that the magicians use an object such as box, table, hat or cloth to transform an object to become another object, a person with blue cloth to different type and colour of clothes. 

from a bird

to become a rabbit

The object that the magicians use to transform an object/ a person to another thing works the same function as function in algebra.

Function (in algebra) works as a machine which can change a 'thing' to become another 'thing'. In the function, there is a rule that enable the function to operate.

Example follows:

Input - Function - Output

       
The concept claims that it is always one output for every input. Two inputs can give the same output but one input cannot have two different outputs.

Algebra: Introduction and Pattern

Algebra

Algebra is a branch of mathematics that uses mathematical statements to describe relationships between things that vary over time. Algebra is about finding the unknown or it is about putting real life problems into equations and then solving them (Rusell, 2010).

There are two patterns in algebra which are repeating and growing.

Repeating pattern refers to a sequence or set which is repeated over and over. Meanwhile, growing pattern is still a repetition of a set or sequence but in each repetition, there is an addition of any attributes e.g. quantity or shape.

There are five processes involved in pattern – recognize, copy, extend, create and translate.  Recognizing a pattern is the process to detect the foundational idea in the pattern.

Copying a pattern refers to the process to detect the foundational idea and re-create, copy or describe a pattern.

When a pattern is extended, the set of elements is expanded to a larger set, and the rule is extended across the larger set.

Example:

In this example, from two block, the blocks extends to become four block, six blocks and eight blocks. 

Create a pattern is the ability to come up with a new pattern using the same foundational idea and retaining the rule.

Translate a pattern refers to the ability to identify the rule and represent the pattern using different elements.

Montessori Math Method

In this video, it is shown how children should be able to count with the presence of material used. Children can see and learn better if the things they learn are tangible. This is parallel with what Piaget has said that children learn best according to their stages. In the pre-operational stage, they need to deal with concrete objects to learn. Thus, it is worth for teachers to consider instigating such materials as number representation in classroom. There are many items that can become representations such as seashells, counters, marbles, etc.





Next, in this video we are introduced with checkered board, a board that helps children to do multiplications of big numbers. Even me myself are impressed of how the material is used to multiply 6425 x 3 in very simple way. Indeed, it takes time but it is effective in the way it can help the children learn Mathematics. However, it is obvious that the students need to master number sense awareness to be able to use this checkered board.






This video tells us how to use objects around us to get our children learn Mathematics. This suits for parents who want to teach pre-number among their children. In fact, teachers can ask their students to bring materials from home in learning Mathematics.


It is interesting to view these videos of Montessori Mathematics Method. Teacher should consider this method to be part of their resources in teaching and learning. After all, teaching Mathematics require teachers to enrich themselves not only with knowledge and skills but also brilliant ideas and suitable materials.

Mathematics in Malaysia: A Reflection.

I started learning Mathematics when I was in Year One, 7 years old. And last time I learned Mathematics was last three ago when I was in Form Five in Upper Secondary School. Mathematics was my obsession throughout my schooling. I always aimed for a good grade in every test or exam or quiz. 

This semester when I am enrolled in Exploration in Mathematics for Early Childhood, I started realizing how different the ‘environment’ of Mathematics classroom in Malaysia and in Australia. I do not have much experiences and exposure to Mathematics classroom in Australia but based on five week interaction in the tutorial I can draw some differences that I found between Malaysia and Australia.

* * * 


When I was in the school, I can remember that my teacher never employ games in the classroom. The only time my friends and I played ‘academic’ games such as Monoply and Snake & Ladder was in the library. 

However here, I am exposed to many kinds of mathematical games that are very engaging, interactive and attracting, at the same time very educational. For example, we played games on ‘division’ in which we have to do a division problem to play the games. 


I believe this is an effective way of teaching mathematics in a way it seems to be relaxed and engaging but at the same time give knowledge on mathematical concepts to the students.


* * * 


It impressed me when I saw local students in my class are able to tell the strategies they are using in coding mathematical problems. Indeed, anyone would give the correct answer but not all can give the strategy they use to attain the answer. 
Personally, at the beginning it is difficult for me to share how I come to an answer for any mathematical problems. 


This suggests that Australian schools are teaching their students the strategies to settle mathematical problems but in Malaysia we are taught using spoon-feeding. 


For example, from primary school, we are expected to memorize multiplication chart from chart 1 until chart 12. Every morning before coming to the class we have to say the multiplication charts beside the classroom.

I always believe that mathematics should be fun. Learning mathematics should be expanding students’ thinking and knowledge. In spite of the fact that mathematical skill is an important literacy in the real world, that mirrors it as a very serious subject; we should give children an opportunity to learn the subject in more interactive and relaxed way. 


All in all, the opportunity to see and experience mathematic classroom in Australia is very meaningful in the way this can be an initiator for us, future teachers, to bring good idea to be implemented in Malaysia soon. 

Using Songs in Mathematics

Who don't love song? Everyone loves to sing songs. Thus, the idea of using songs in teaching Mathematics should be taken into serious consideration.


This is an example of a song on introducing numbers for beginner students.

* * *


With the advent of internet and computer, resource like songs become available for teachers to use in classroom. Websites such as YouTube and http://www.songsforteaching.com/mathsongs.htm offer variety of songs that can support teachers to achieve the objectives of the lesson.


Why Do We use Songs? 


Simple, students come to school with the knowledge of songs like 'Mary Had A Little lamb' or ' Twinkle Twinkle Little Star'. Imagine if the students come to school with the acquisition of Mathematics concept by all their heart through songs. Can you see the effects songs can bring to students' life?


In fact, musics and songs get students to partcipate, adding another levels of engagement among students. Obviously, they need to memorize the lyric for them to be able to sing and 'enjoy' the tune. It is fine for them to sing if the teacher ask any questions that need them to recall the yric of the song. For example, if they have memorize a song on number one to ten. Then it is okay for them to sing the song to recall their memory.


However, it is important for teachers to note that the objectives of the lesson are still regarded as the main agenda, using songs as a tool to help the students acquire mathematical concepts.